

Reactor Containment Field

The reaction plasma reaches such extreme temperatures that no physical material could survive direct contact with for any practical duration. Instead, the plasma is contained within powerful magnetic fields generated by a combination of superconducting field generators.
The containment field plays a key role in maintaining the reaction by keeping the plasma under pressure and isolated from physical reactor components that could act as contaminants. However, the field exerts its own influence on plasma characteristics that must be carefully managed to prevent detrimental impacts to plasma flow patterns.
Containment Theory
Magnetic fields exert a Lorentz force on charged particles in plasma that is perpendicular to the magnetic field lines, effectively confining the plasma isotopes within the magnetic field. A series of overlapping magnetic fields create a torus-shaped space within which the confined plasma circulates endlessly.
The helium atoms and high-energy neutronic particles that are a byproduct of the reaction process have no charge and so are unaffected by the containment field. If the reaction plasma is maintained at a sufficient density the neutronic particles will impart energy to help sustain the reaction via collision with fuel isotopes, before eventually escaping the containment field and being stopped by the reactor wall. Helium atoms, in the form of ash, will drift to the bottom of the reaction chamber for removal by the divertor assembly.
The geometry of the main containment field is modified by secondary fields to ensure magnetic field lines promote efficient plasma flows (a principle known as magentohydrodynamics). Poorly optimised field lines can result in localised temperature variances within the plasma or result in plasma drifting out of containment, releasing damaging high-energy bursts as it does so.
Containment Field Geometry
The reactor containment field is generated by a series of powerful superconducting electromagnet assemblies, the toroidal field generators. The strength of the magnetic fields generated keeps the plasma under sufficient pressure to enable a self-sustaining reaction to be maintained.
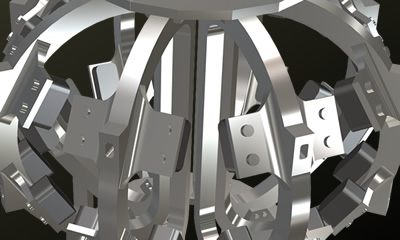 The toroidal field generators create a solenoidal vector field, so called because the field lines (points of similar field intensity) for this kind of field neither start nor end: magnetic field lines continue through the magnet from one pole back to the other, so that a field line either wraps around to form a closed curve or extends to infinity.
The toroidal field generators create a solenoidal vector field, so called because the field lines (points of similar field intensity) for this kind of field neither start nor end: magnetic field lines continue through the magnet from one pole back to the other, so that a field line either wraps around to form a closed curve or extends to infinity.
Like all solenoidal vector fields, the active field line would normally trace a parabolic line, creating an open-ended magnetic container. A paired field generator of equivalent strength is required to generate a mirrored field, which closes the magnetic container. The interaction between the two fields alters the field lines of both where they meet, pulling both towards each other. The combined field lines form a mild parabolic curve.
A pair of field generators aligned in this way creates a reactor segment. There are eight toroidal generators, with each pair of generators creating a segment, for eight reactor segments in total.
Active Field Plane
Magnetic field strength can be measured in a number of ways. Magnetic flux density measures the overall (peak) output of magnetic field generators and describes the effect of the magnetic field on charged particles (such as plasma). The unit of measurement is the tesla.
The magnetic flux density (or overall strength of the magnetic field) reduces with distance from the field generator and so a more useful measure for the purposes of plasma containment is magnetic field intensity. This measures the specific impact of the lorentz force on charged particles, with the unit of measurement described in amperes/metre (A/m). Identical field intensity measured at a series of different points along the field generator’s profile describes a magnetic field plane. The topography lines on geographical maps or isobars on weather maps represent two-dimensional analogs of this concept and in fact on most instrumentation a selected cross-section of the plane is represented by a magnetic field line to reduce visual complexity.
The point inside the containment field where magnetic field intensity is at the minimum necessary to contain the plasma represents the active field plane. As this is where the containment field interacts with the plasma, it is the active field plane that is monitored for containment purposes.
As described above, field generators work in pairs to create the containment field for one reactor segment. The active field planes of each generator meet at the mean distance between the generators, effectively forming one continuous active field plane for the segment.
Containment Field Pitch
The active field plane is essentially a function of distance from a given point on the field generator to the centre of the area enclosed by the generator. There is another axis to be considered, which is the distance between field generators. As field intensity diminishes with progression along this axis, the active field plane naturally shifts towards the outer edge of the reactor. 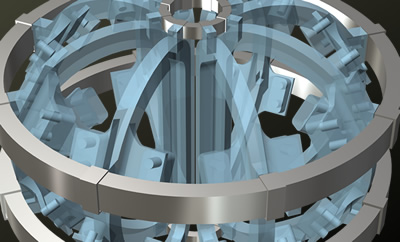 The curve described by this shift is referred to as the containment field pitch.
The curve described by this shift is referred to as the containment field pitch.
An unmediated containment field pitch creates a relatively deep curve which has a constrictive effect on plasma flow by creating a magnetic bottle, trapping significant portions of plasma within the reactor segment. This adversely affects smooth plasma flow and creates temperature differentials between segments, all of which dramatically increases the occurrence of magnetohydrodynamic instabilities.
In order to compensate, the containment field pitch is shaped using secondary magnetic fields, generated by poloidal field generators placed perpendicular to the toroidal generators. Magnetic fields produced by these generators interact with the main containment field to modify the containment field pitch, reducing plasma flow constriction between segments.
Magnetohydrodynamic Drift Compensation
The reactor’s design impacts on plasma magnetohydrodynamic characteristics in a number of ways which must be compensated for to ensure the efficiency of the reaction. Like all magnetic fields, the containment field introduces an electrical charge into the plasma, giving the plasma isotopes their own magnetic field which distorts plasma flow.
In addition, the reactor’s toroidal shape means the positioning of field generators differs between the inner and outer edge of the reactor, resulting in a difference in containment field impact on plasma isotopes.
To compensate, a central solenoid within the core of the reactor supplies an inductive drive current into the reaction plasma. This drive current must be precisely balanced with the containment field’s flux density and the countervailing impact of the different forms of magnetohydrodynamic drift in order to ensure optimal plasma flow characteristics.
More information on this is included in the reactor plasma article.
Containment Field Management
Effective management of the containment field requires an optimal balance between the size of the reaction and the pressure under which it is maintained. The area within the active field plane describes the volume within which the reaction takes place and is referred to as the effective reaction volume (ERV).
The reactor is optimised to run with a specified ERV range and increasing the ERV does not necessarily increase the output of the reactor. An appropriate level of fuel plasma as a proportion of the ERV must be maintained in order that the plasma is under sufficient pressure to sustain the reaction. Independently of the plasma levels, the size of the ERV must also be maintained in proportion to the magnetic flux density to prevent unacceptable variances in flux density between the edge and the centre of the reaction.
Magnetic Flux Density Range
Even at the same plasma density, the strength of the magnetic field lines at the core of the plasma would be reduced in proportion to the outward shift in the active field line (because the strength of the magnetic field reduces further from the field generator). The greater range in field strength between the centre and outer edge of the ERV caused by the increased distance between the two would cause a wider variation in the effect of the magnetic field on plasma flow characteristics. This wider variation reduces the ability of a drive current to correct flow characteristics and will have the effect of increasing magnetohydrodynamic instability at the core - instabilities which would migrate out from the core and disrupt overall plasma flow - reducing the reaction temperature (and therefore the efficiency of the reactor).








